Objects, as long in simple translational motion, can be treated as point particles. However, real movement is much more complicated: objects rotate, vibrate, etc.
Yet even during more complicated motion, there is a single point within the object in which we can treat the object as a point. This is the Center of Mass
When we fix our attention on the center of mass, we have a place to start our analysis.
For example, when we throw a baton:
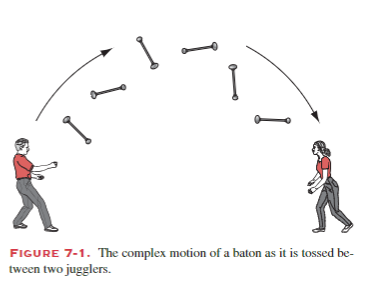
We can use its center of mass to find its trajectory. However, we won’t be able to calculate the other points as simply.
Separate the problem into a system and environment, where we define the system as any number of particles in order to simplify the problem. Interactions within the system are internal forces and outside are external forces.
For our baton, our system is the two particles (one big and one small) and the rod connecting them and our external forces are gravity and the normal force.
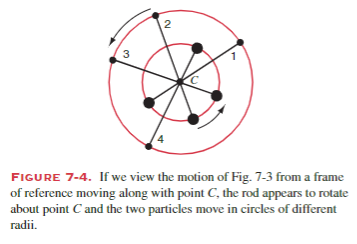
Viewing our system, the motion is a simple rotation.
Baton Example for a System of Particles Conservation of Momentum in a System of Particles Systems of Variable Mass